Ask Professor Puzzler
Do you have a question you would like to ask Professor Puzzler? Click here to ask your question!
Today's question is a probability question from Sue. Her question is about how to calculate the probability of winning a game at least a certain number of times.
Let's say the probability of Sue winning Fraction Concentration against the computer is 3/4. If she plays 8 times, how do you calculate the probability that she'll win at least 6 times?
If she wins at least six times, that means she could win 6, 7, or 8 times.
So we're going to have to work out the probability that she wins exactly 6 times, the probability that she'll win exactly 7 times, and the probability that she'll win exactly 8 times. Once we've found those three probabilities, we add them together to get the total probability.
Note that in the equations below, 3/4 is the probability of a win, and 1/4 is the probability of a loss (since 1 - 3/4 = 1/4).
Six wins: (3/4)^6 x (1/4)^2
Seven wins: (3/4)^7 x (1/4)
Eight wins: (3/4)^8
Now, depending on who your teacher is, and whether or not they like to torture you with fractions, you'll either have an ugly fraction, or a decimal answer. I came up with approximately 0.1446.
Now that you know the process, you can fill in any numbers you like. Make the probiblity of winning 0.05. Make the number of games 20. Whatever you like.
Helpful tip: Suppose you played 20 games, and wanted the probability of winning at least 5. Using this method, you'd have to find the probability of winning 5, 6, 7, 8,...18, 19, or 20 games. Not pretty.
So instead, Find the probability of LOSING no more than 4. That's a much easier probability, and it comes out to the same thing!
Happy computing!
Professor Puzzler
Dear Professor Puzzler,
I think your "What is Wrong" game is obnoxious. Who cares if someone gets their spelling wrong, or uses an apostrophe when they weren't supposed to?
Annoyed in Alaska
Hi Annoyed,
You're not alone in feeling that way. In fact, a few months ago, the Wall Street Journal website had an article in which the writer said (in essence), "Language is evolving. Yay! Everyone who gets in the way of language evolution is a pedantic grammar snob."
Frankly, it was a poorly thought out article, which left a giant hole in its logic. The hole is between "language is evolving" and "Yay!"
The author accepted it as a given that since language is evolving, that must be a good thing. There was no research, no exploration, no evaluation of the question, "Is language evolution a good thing?" And if you're going to be annoyed with the "What is Wrong" game, you need to answer that question.
What do I think?
I think that some language evolution is good. For example, what would we do without the word "computer" added to our vocabulary? New technology requires new vocabulary.
But there is a bad side to language evolution. I've been in a small country in Africa where from one end of the country to the other, even though they speak the same language, they can't understand each other. Why? Because in that country, language evolved unchecked in isolation, until the dialects defied mutual comprehension. It was a strange thing to realize that people who spoke the same language had to resort to using the trade language (French) to communicate with each other!
Fortunately, in our society, with written language and easy global communication, we're less likely to have isolated language evolution. But there are still concerns to be considered.
Pick up a King James Bible. Or the complete works of Shakespeare. How easy are they to understand? Not terribly. That's a result of language evolution over a few centuries. Someone once said that the English speaking world is the only part of the world that doesn't get to read Shakespeare in our own language, because the English of his day is not our language.
The more quickly language evolves, the more quickly the great works of literature become inaccessible without translation.
Is language evolving? Yes it is. Can we stop that evolution? Probably not. Should we try to stop that evolution? Maybe not. But I think that, at the very least, we should work to keep that evolution to a slow pace.
Ever seen a car on glare ice? Watch the way it slides around uncontrolled? Friction is needed to keep things running smoothly. And language evolution needs friction too, to prevent the sort of uncontrolled slippage that made linguistics such a nightmare in that African country I mentioned.
Every English teacher who says, "You need an apostrophe there," or "You should be using the word they're instead of their," or "Your subject and verb don't agree; please fix that!" is applying friction to linguistic evolution. And I say, "God bless them for it!"
So, that's why I am such an obnoxious, cranky old geezer when it comes to grammar, spelling, and word usage.
Professor Puzzler
Dear Professor Puzzler,
Can you explain to me the difference between correlation and causation? Is there a difference?
Thanks,
Martin
Hi Martin,
Correlation is a strong link between two sets of data. For example, suppose I created a graph of electricity usage by month from January to December, and then, on top of that graph, I superimposed another graph of ice cream consumption per person by month, you might find a correlation between the two sets of data. As ice cream consumption increases, so does electricity usage. Similiarly, as ice cream consumption decreases, electricity usage does also. We say there is a correlation or link, between those two data sets. We haven't made any claims about the reason for that link; we have simply acknowledged that the link exists.
Causation is a word that is used to indicate that one thing causes another. Causation is NOT the same as correlation. Consider the example of the ice cream and the energy usage. Does eating ice cream cause you to use more electricity? Of course not! Does using electricty cause you to eat ice cream? No! Those are both silly ideas!
So what's going on here? It's simple: as temperatures increase, people are more eager to eat ice cream, because it cools them down. But at the same time, people are running air conditioners more, and their refrigerators are working harder because of the heat. Both the ice cream consumption and the energy consumption are a result of the changing temperatures from one month to the next.
This is why it's very important to understand that correlation does not imply causation. Just because two things have a correlation does not mean that one causes the other!
Here's another example. Take a look at the chart below. It's used as proof that autism is caused by the use of herbicides on crops:
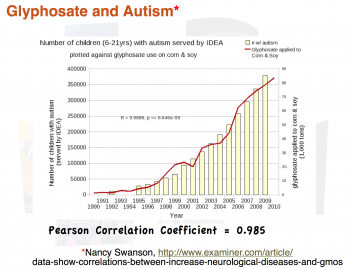
It shows a very definite correlation between herbicide use and autism. Later on we'll tear this graph apart, because it is one of the most attrociously unscientific graphs I've ever seen, and the fact that it's linked from the home page of an MIT researcher is downright embarrassing. But we'll get to that a bit later.
Now that you've looked at this graph and drawn the obvious conclusion: Herbicide causes autism, take a look at the next graph, which shows another, quite surprising correlation.

Yes, that's right - you're reading that graph correctly. There is a very clear correlation between purchase of organic foods and autism. The conclusion is very clear: Organic foods cause autism!
I'm sorry, you can't have it both ways. If the first graph (which is merely a correlation) is proof of causation, than so is the second one.
The real challenge is this: if you can't eat chemically treated crops, and you can't eat organic crops, how do you avoid autism without starving to death?
The Embarassing Glyphosate and Autism Graph

I promised we'd tear apart that herbicide/autism graph, and we will. This graph has been shared on the home page of an MIT researcher (whose post graduate degrees are in Electrical Engineering and Computer Science). Never mind the fact that she probably doesn't have any business meddling in biology related stuff, if you have a masters degree or a Ph.D. in any subject area, you know enough to realize that this chart is horrifically nonsensical. For crying out loud, my high school science students probably know enough to realize how dumb this chart is.
So, is this graph really so bad? And if so, how? Oh, let me count the ways...
- The graph uses total numbers of autism cases instead of autism cases per capita, which means it does not take into account population increase (which was significant between 1990 and 2010).
- The graph shows only autistic children served by IDEA (Individuals with Disabilities Education Act). What's wrong with that? Oh, I don't know, maybe the fact that IDEA started serving autistic children in 1990, which means the first few years of that graph are ramped up because IDEA was ramping up.
- The graph does not take into account the fact that diagnosis rates have significantly increased over that time interval; more and more children who used to be diagnosed with mental retardation are now being diagnosed as autism spectrum.
- The graph shows total gallons of herbicide, without any reference to gallons per acre, or number of acres of cultivated land.
- One chart represents USA numbers (autistic children served by IDEA) while the other chart is worldwide (do you really think we don't export significant amounts of corn and soy? Check the USDA site if you doubt it!) If you're comparing US numbers with global numbers, you're not just comparing apples with oranges, you're comparing apples with harmonicas. Or some equally absurd comparison.
- The correlation goes even further out the window if you attempt to limit your gallons of herbicide to ONLY crops used for human consumption. Based on a quick scan of USDA and NCGA websites, it appears that the amount of corn used for ethanol is from 20% to 40% of the entire corn harvest, and that number is rapidly increasing.
Are there more problems with this graph? Oh, undoubtedly! Those are just the ones I came up with off the top of my head, without doing any serious research. And yet, ironically, the "researcher" who shares this on her home page has the audacity to whine about scientific journals that have unfair standards of statistical analysis.
So, Martin, you probably got more than you bargained for - this turned into a little rant (hope you don't mind). But I hope it'll help you remember that just because two things have a correlation doesn't mean that one caused the other!
Professor Puzzler
P.S. If you're wondering why I didn't rip the second chart to shreds, it's because no one actually believes the second one; it was created entirely to prove a point. The organic-food chart (along with a diabetes chart) can be found here.
Question
Dear Professor Puzzler,
What is the difference between the words "compliment" and "complement"? Do they mean the same thing?
From, Lizzie in Sacramento
Answer
Dear Lizzie,
The word "compliment" is what you call it when someone says something nice about someone. The word "complement" is used to describle something that completes something else. In mathematics, "complementary" is used to describe angles that add up to a right angle when put together. You may also hear the term "complimentary" used to describe something, and that generally means it is something that is free, in other words, given "with our compliments."
- Megan gave me a very nice compliment about the color of my earrings.
- My spouse and I complement each other very well.
- We greatly enjoyed the complimentary breakfast at the hotel this morning.
Sincerely,
Professor Puzzler
Question
What's the difference between an adjective and an adverb?
Wallace
Answer
Hi Wallace,
First, let's talk about how adjectives and adverbs are alike, then we can talk about how they're different. Adjectives and adverbs are parts of speech, and they are both words that are used to describe other words. For example, if you say "That's a good question," good is a describing word, so it's either an adjective or an adverb. Can you find the describing words in the following sentences?
- I ran very slowly.
- I saw a blue balloon.
- The extraordinarily ugly monster ate the burnt toast.
How did you do? In the first sentence, "slowly" is a describing word; it describes how you were running, and "very" is also a describing word, because it helps to explain just how slowly you were running. In the second sentence, "blue" is a describing word, and it describes the balloon. In the third sentence, there are three describing words: "extraordinarily," "ugly," and "burnt."
Now let's talk about how adjectives and adverbs are different. The difference can be described quite easily as follows: if a word is describing a noun, it is an adjective. If it's describing anything else (a verb, an adjective, or another adverb), then it's an adverb.
So, with that in mind, can you go back through those three sentences above and determine which are adjectives, and which are adverbs?
Here are the answers:
- adjectives: blue, ugly, burnt
- adverbs: very, slowly, extraordinarily
By the way, sometimes people will tell you that you can recognize adverbs because they end in "ly," but that's not always true. For example, in this sentence, always is an adverb, but it definitely doesn't end in "ly!"
I hope that helps, and thanks for asking!
Professor Puzzler

